First order kinetics
n., plural:
[fɝst ˈɔɹdɚ kɪˈnɛt.ɪks]
Definition: A chemical reaction where the rate of reaction is directly proportional to the concentration of a single reactant.
Table of Contents
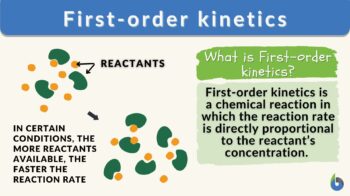
What is a First-Order Kinetics (First-Order Reaction)?
First-order kinetics refers to a reaction wherein the overall rate behavior of a reaction follows a first-order rate equation, which is rate = k[A], where [A] represents the concentration of reactant A, and k is the rate constant. As it follows the first-order rate equation, the rate of reaction is, thus, directly proportional to the concentration of only one reactant. There are three fundamental concepts comprising its definition that we need to dig deeper into for us to understand the term. These are reactions, kinetics, and first-order reaction. Let’s find out what these concepts are and how they are related to first-order kinetics.
Reactions
Let’s understand what a reaction entails in chemistry. A chemical reaction is a process that transforms one or more substances (called reactants) into one or more new substances (called products). How such a transformation process proceeds and how fast or slow (speed) will rely on various factors. Examples of these factors are temperature, the presence of catalysts, and the concentration of the reactant(s) among many other reaction conditions.
Various factors will determine the speed at which reactants are consumed and transformed into products during the course of the reaction. Such a speed is referred to as the rate of the chemical reaction. It is determined by the change in the concentration of a reactant or product divided by the corresponding change in time, typically expressed in units such as moles per liter per second (mol/L/s) or moles per liter per minute (mol/L/min). That is:
rate = Molarity (M)/second (s)
Kinetics
A branch of chemistry that specifically focuses on the rates of chemical reactions is called kinetics. It aims to understand the chemical reaction rate dynamics and mechanisms as it looks into the following:
- How the reactants transform into products as their molecules collide and their chemical bonds break and form
- The pathways they thread, and
- The energy that drives the transformation
As a science field, kinetics speaks of reaction rates rooted in mathematical analysis and experimental observations. Thus, kinetics studies how quickly or slowly chemical reactions occur and what could have speeded them up or slowed them down. In learning kinetics, you will come across fundamental concepts, such as:
- Reaction rate laws (or rate equations): The mathematical expressions describing the relationship between chemical reaction rate(s) and reactant concentration(s). The rate laws include the reaction rate constant and the reaction orders.
- Reaction rate constant: The proportionality constant specific to the reaction.
- Reaction orders: The mathematical relationship between the reaction rate and the concentration(s) of reactant(s) indicating how reactant concentration(s) influence the rate of a chemical reaction. The common reaction orders are zero-order, first-order, and second-order reactions.
First-Order Reaction
The reaction order tells how the rate of chemical reaction (i.e., how fast or slow the reaction would proceed) depends on the concentrations of one or more reactants. It will answer questions such as this:
“Will the reaction go faster if the amount of the reactant(s) is increased? … or will it stay the same?”
Take note that some reactions tend to speed up with the increasing amount of the reactant(s) while other reactions will proceed at about the same rate. Thus, we have terms such as zero-order, first-order, or second-order.
So having mentioned these reaction orders, what, then, is chemical kinetics first-order reaction? How does it differ from the other orders?
A first-order reaction is when the rate of reaction is directly proportional to the concentration of only one reactant. This means the reaction rate would depend on the first power of the concentration of the reactant. Therefore, the first-order reaction may be represented by this equation:
A → Products
where “A” represents the reactant, and “Products” denote the resulting products of the reaction.
The rate equation for a first-order reaction, in turn, may be written as this:
First-order rate law (or the first-order kinetics equation): rate = k[A]
where [A] represents the concentration of the reactant A, and k is the rate constant. The rate constant is specific to each reaction and depends on factors, such as temperature, the presence of catalysts, and molecular collisions.
First-order vs. Zero-order and Second-order reactions
Apart from the first-order reaction, there are two other orders: zero-order and second-order. They differ in how their rate of reaction depends on the concentration of their reactant(s).
Take note:
» In zero-order reaction, its rate does not depend on the reactant concentration
» In second-order reaction, the rate depends on the reactant concentration. But as opposed to the first-order being raised to the power of one, the rate will increase/decrease to the power of two.
Here is an example:
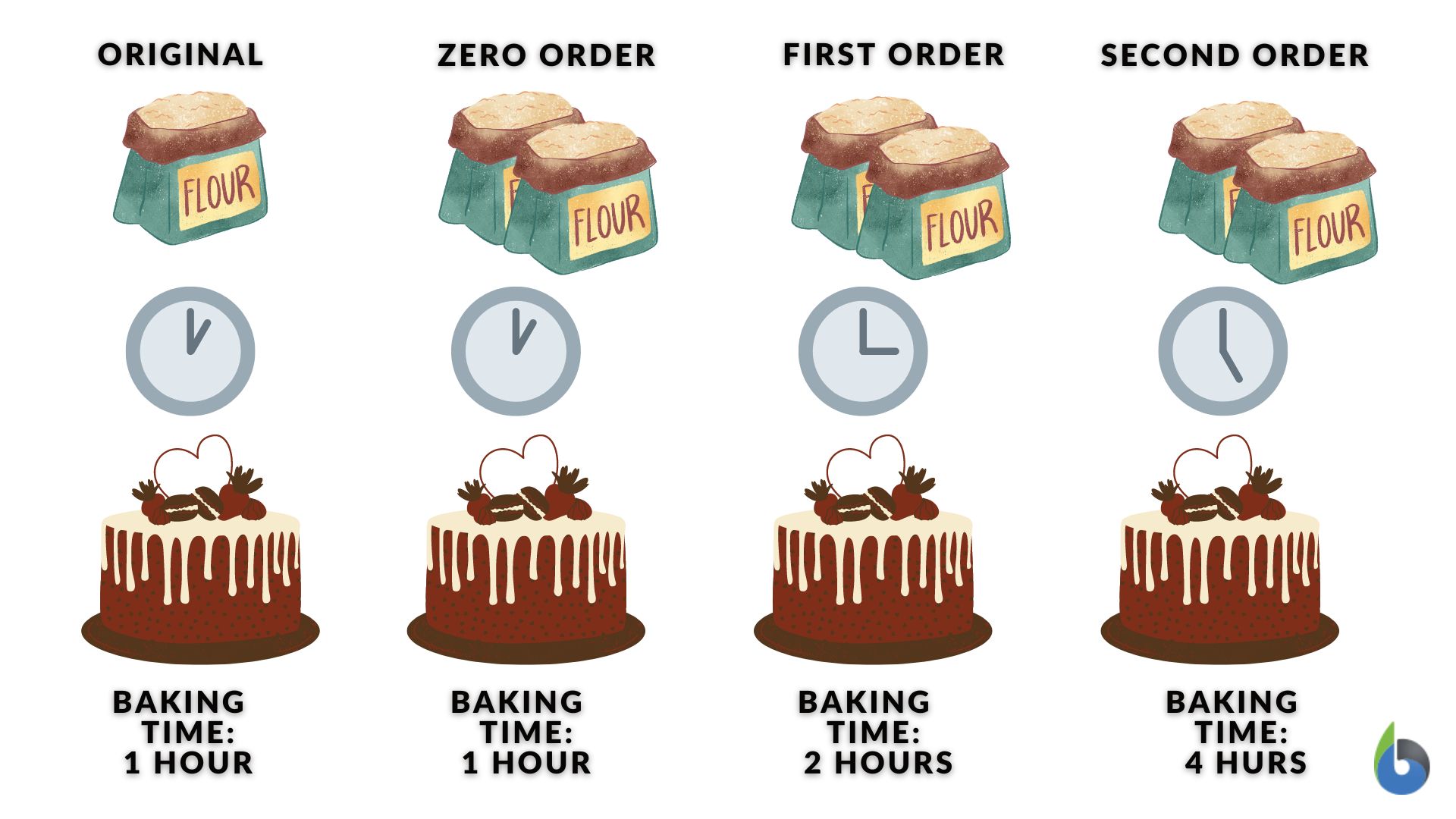
When baking, will the amount of flour ingredient (reactant) in the recipe affect baking time (reaction rate)?
» Zero order: when a recipe is supposedly in zero order, this means that the baking time will remain the same whether you put more or less flour. The baking time (reaction rate) will not be affected by the amount of flour (concentration of the reactant).
» First order: when a recipe is supposedly in the first order, this means that the baking time will depend on the concentration of the flour. Increasing the amount of flour means you have to increase the baking time and decreasing the flour means you have to reduce the baking time. The amount of flour in the recipe, thus, directly affects the cooking time. So while the other ingredients are kept the same but the flour is doubled, the baking time is also doubled. This “reaction” is, therefore, a first-order with respect to the reactant (flour), where [A] = 2, then rate = 2
» Second order: when a recipe is supposedly in the second order, this means that the effect on the baking time is significantly greater than it is in the first order. Doubling the amount of flour will quadruple the baking time (increase by a factor of four, instead of by a factor of two in first order). Decreasing the amount of flour by half will, in turn, decrease the baking time to one-fourth of the original time. Baking time depends on the square (or product) of the amount of flour. This “reaction” is, therefore, a second-order with respect to the reactant (flour), where [A] = 2, then rate = 4
Therefore, the reaction rate equations are specifically as follows:
» Zero order: rate = k
» First order: rate = k[A]
» Second order: rate = k[A]2 (or rate = k[A][B])
More differences between these three reaction orders are summarized in the table below:
Table 1: Three Common Reaction Orders | |||
|---|---|---|---|
| Features | Zero-order | First-order | Second-order |
| Dependency on the reactant concentration | The rate of reaction does not depend on the concentration of the reactants. | The rate of reaction depends on the concentration of one reactant. | The rate of reaction depends (1) on the doubled concentration of one reactant or (2) on the product of the concentrations of two reactants. |
| Reaction rate formula or equation | The zero-order reaction equation is expressed as: rate = k where k is the zero-order rate constant | The first-order reaction equation is expressed as: rate = k[A] where [A] represents the concentration of the reactant and k is the first-order rate constant | The second-order reaction equation is expressed in either of these two ways: (1) rate = k[A]2 (2) rate = k[A][B] where [A] and [B] represent the concentrations of the reactants and k is the second-order rate constant |
| Rate equation | R = constant | R= k[A] | R = k[A]2 or R = k[A] [B] |
| Concentration | [A] = kt | [A] = [A]o exp (-kt) | 1/[A] = 1/[A]o + kt |
| Half-life | = [A]o/2k | = 0.693/k | = 1/k[A]o |
| Implication (with respect to the concentration of the reactant) | In zero-order reactions, the rate will still be the same even while increasing amounts of the reactant. | In first-order reactions, the rate will be faster with the increasing amounts of the reactant, e.g., the rate doubles when the reactant concentration is doubled | In second-order reactions, the rate will be even faster (quadruples) with the doubling amounts of the reactant. |
Data Source: Maria Victoria Gonzaga of Biology Online
Relevance in Biology
In biology, kinetics is relevant when studying the rates of biological reactions and processes, especially at the molecular level. It will find answers as to how biological molecules, such as enzymes, proteins, and substrates, would interact in such a reaction and what possible factors would affect the speed and efficiency of their interactions. Thus, in biology, the principles of kinetics are important in understanding:
- Enzymatic reactions
- Molecular binding and dissociation
- Signaling pathways
- Drug interactions.
First-order kinetics may, therefore, be defined in biology as follows …
First-order kinetics is when the rate of a reaction follows a first-order equation, where the reaction rate is directly proportional to the concentration of a single reactant. The rate equation is represented as the first-order reaction formula, i.e., rate = k[A], where [A] represents the concentration of the reactant and k is the rate constant. As the concentration of the reactant decreases over time, the reaction rate decreases logarithmically. An example of a reaction in a first-order is the decomposition of N2O5 (i.e., N2O5 → 2NO2 + ½ O2) because the rate of reaction depends on the concentration of N2O5 raised to the first power.
Synonym: unimolecular kinetics
Compare: second-order kinetics
First-Order Reaction vs. First-Order Kinetics
Perhaps, you noticed the terms “first-order reaction” and “first-order kinetics” with their respective definitions above. Have you noticed how similar these two terms are? Are they the same? These terms are often used interchangeably depending on the context of the discussion. They are very much related and have the same context, i.e., that which indicates a reaction follows a first-order rate equation.
Should there be a difference in their usage, it would only be subtle. The term “first-order kinetics” particularly refers to the overall rate behavior of a reaction with respect to the concentration of the reactant. “First-order reaction”, in turn, is about mathematically describing each instance as to how the reactant, with respect to its own speed, contributed to the overall rate.
Take for instance a group of individuals working to complete a puzzle. As more people there are, the faster the puzzle will be completed. Zooming into the individual’s speed, everyone contributed to the overall rate needed to complete the puzzle quickly.
Another example is when removing a pile of snow. The overall speed will depend on the people removing the snow pile. The more people scooping, the faster the removal of the snow pile. Doubling the number of individuals scooping the snow, the rate also doubles, thus, the less time needed to finish the task. (First-order kinetics)
Now, imagine you being one of them. The speed at which you remove the snow will directly depend on the amount of snow. At first, the more snow there is, the faster you are. That’s because there will be more snow with each scoop. However, as the pile shrinks, your speed decreases as the amount of snow gets smaller with each scoop. In this scenario, the pile of snow represents the concentration of the reactant and the rate of shoveling is the rate of the reaction. So just like in a first-order reaction, the more snow (higher concentration), the faster you are in removing them (faster reaction rate). As the snow pile shrinks (lower concentration), the slower you are in removing them (slower reaction rate). (First-order reaction)

More orders, anyone?!
Question: Are there kinetic or chemical reaction orders higher than the second order?
Yes, there is!
There are other higher orders apart from the three mentioned above. For instance, there is the third-order reaction, where the rate is proportional to the cube of the concentration of a single reactant (increased by eight-fold), or rate = k[A]3, the fourth-order reaction, to the fourth power of the concentration of a single reactant, i.e., rate = k[A]4) (increased by 16-fold), and so on… These are not as common though as the first three mentioned above. Higher-order reactions often involve a rather complex reaction mechanism and interactions.
And with that, you might probably be wondering, “Are there lower reaction orders?”
Yes. Albeit not common as well, there are fractional reaction orders or negative reaction orders. Negative reaction order would implicate that a reaction rate would decrease rather than increase as the reactant concentration is increased, which in a biological process would appertain to an inhibitory reaction activity.
Examples of First-Order Reactions
In biology, here are a few of the many first-order reaction examples in biological processes:
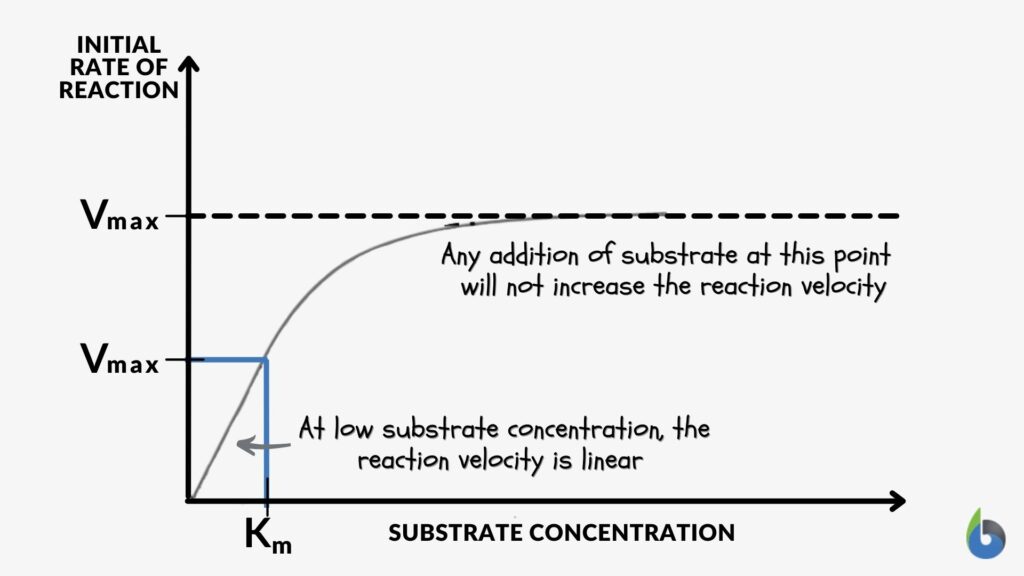
- Enzyme kinetics: Enzymes are vital as they speed up reactions until a saturation point is reached. Therefore, many of these enzymes would be in the first order. This enzyme-catalyzed reaction that follows a single-substrate enzyme reaction is, in fact, a classic example where Michaelis-Menten kinetics can be applied where the formula is given by: Velocity (the rate of the reaction catalyzed by the enzyme) = (Vmax [S]) / (Km + [S]), where Vmax is the maximum rate at which the enzyme is fully saturated with the substrate, Km is the Michaelis-Menten constant, and [S] is the substrate concentration.

Figure 3: Graph showing the relationship between the reaction rate and substrate concentration. Image prepared by Maria Victoria Gonzaga for Biology Online. Examples of enzyme-catalyzed reactions are protein degradation by proteolytic enzymes, conversion of precursor molecules, and other metabolic pathways.
- Radioactive decay: The rate of the degradation of radioactive isotopes, such as carbon-14 (C-14) is in the first order. The rate of their decay is directly proportional to their concentration.
- Pharmacokinetics: Drug metabolism/elimination often involves a first-order reaction where the removal of the drug from the body is proportional to its concentration.
Rate Laws
Reaction rates typically decrease with time as the reactants are used during the transformation (or conversion) into products. The relationships between the reactant rates and the reactant concentrations are expressed mathematically and empirically as rate laws.
Two perspectives to write rate laws:
- Differential rate law: where the reaction rate is expressed as changes in the concentration of the reactant(s) (Δ[R]) over a specific time interval (Δt)
- Integrated rate law: where the reaction rate is expressed as the initial concentration ([R]0) and the measured concentration of one or more reactants ([R]) after a given amount of time (t)
Differential Rate Law For A First-Order Reaction
Because a first-order reaction is directly proportional to the concentration of the reactant, the differential rate law can be specifically applied to it. The term “differential” here refers to the differentiation, which in a mathematical operation, pertains to calculating the rate of change of a function with respect to an independent variable. Thus, in this case, what is “differentiated” is the concentration of the reactant at a specific point in time (which is denoted as Δt).
The differential rate law indicates the rate of change of the reactant concentration over specific time intervals. With this, the rate of the reaction can be expressed in terms of both the reactant concentration and time. Calculating the differentiation of the reactant concentration over time would, therefore, mean calculating the instantaneous rate of change by deriving the concentration of the reactant with respect to time.
The differential rate law equation for a first-order reaction (i.e., A → Products) is, thus, given by:
R = -Δ[A]/Δt = k[A]
where [A] is the concentration of the reactant A at a given time, t is the time, and k is the rate constant specific to the reaction. It is negative because it indicates that the concentration of A decreases over time as it is consumed in a forward reaction. It may also mean that the reaction rate decreases exponentially over time as the reactant concentration decreases.
Integrated Rate Law For A First-Order Reaction
For instance, you’d like to know how the concentration of the reactant changes with time as the reaction progresses… Or, you might want to know how long it takes for a concentration to drop to a certain amount. In this case, you may want to apply the integrated law.
The integrated rate law equation provides the mathematical relationship between the initial concentration of the reactant ([A]0), the concentration at a given time ([A]), the rate constant (k), and the elapsed time (t). The integrated rate law can be derived from the differential rate law for a first-order reaction. [The derivative of ln(1+x) with respect to x is d/dx ln(1+x) = 1 / (1+x)]. The first-order integrated rate law can therefore be expressed as:
ln [A]t / ln [A]₀ = -kt
Where,
the natural logarithm (ln) denotes the ratio of the reactant concentration at a specific time,
[A] is the concentration of the reactant A at a given time,
[A]0 is the initial concentration of A,
k is the rate constant specific to a reaction, and
t represents time
The negative rate constant•time (-k t) indicates that the concentration of the reactant decays or decreases over time as the reaction proceeds.
Given the initial concentration and rate constant, this formula helps determine the concentration of the reactant at any given time in a first-order reaction. It gives insights into the decay or depletion of reactants as the reaction proceeds. And so, if you want to know the concentration of the reactant at any given time for an overall first-order reaction, you may use this formula:
ln [A]t = -kt + ln [A]₀
Example: Rate Law for the First-Order Reaction
Let’s take the following reaction as an example. Here, (two molecules of) hydrogen peroxide (H2O2) decompose into (two) water molecules and (one) dioxygen molecule:
Decomposition of hydrogen peroxide: 2 H2O2 → 2 H2O + O2
Question: What does it mean if this is in the first-order reaction?
Answer: If this chemical reaction is in the first order, it means the rate of the reaction is directly proportional to the concentration of hydrogen peroxide. In other words, if the concentration of hydrogen peroxide is doubled, the reaction rate will also double.
Question: What is the rate law of this reaction if this is in the first order?
Answer: The rate equation for this first-order reaction is given by: rate = k[H2O2].
Question: How will you be able to determine if the reaction is in the first order?
The order of the reaction will have to be determined experimentally. The concentration of each reactant is raised to an exponent reflecting the reaction order (not related to the stoichiometric coefficient, which, in this example, is “2”).
By using initial rates data, reaction trials are run several times while varying the initial concentration of the reactant(s) one at a time so that the effect can be empirically attributable to that particular reactant.
Look at the Figure below and answer the review question below:
Question: Given these experimental data, does this reaction indicate it is a first-order reaction? Why?

Answer: Yes, the example data indicate the reaction is first order with respect to H2O2. That’s because when the molar concentration of hydrogen peroxide is doubled from 1.0 M to 2.0 M, the reaction rate also doubled (from -0.05 M/s to -0.10 M/s).
Graphical Representation Of A First-Order Reaction

The above Figure shows the first-order reaction graphs. The graph on the left shows the concentration vs. time whereas the graph on the right shows the natural logarithm of concentration vs. time.
Reactant Concentration versus Time (Decay Curve)
By plotting the reactant concentration on the y-axis and time on the x-axis, the graph will show a decay curve. At the start, the amount of the reactant is high initially and will gradually decrease as the reaction proceeds. The reactant concentration decreases exponentially over time until the curve approaches zero albeit asymptotically (as it never reaches it completely). The shape of the decay curve will depend on the specific rate constant equation (k) and the initial concentration of the reactant.
Natural Logarithm of Concentration versus Time
With the integrated rate laws, the data can be plotted in y=mx + b (linear plot), where y = ln [A]t, m = -k, x = t, b = ln [A]0. Thus, plotting the natural log of the reactant concentration versus time for a first-order reaction gives us a straight line. This is essential if you want to know the overall reaction order via graphical analysis as the resulting graph will show a linear relationship with a negative slope.
The graphical representation of the first-order reaction helps to visualize how the reactant will decay over time and understand first-order degradation kinetics (e.g., first-order enzyme kinetics).
Half-Life Of A First-Order Reaction
As the name implies, a half-life of a first-order reaction pertains to the time it takes for half of the reactant to be transformed or used up. In other words, the half-life (t½) is the time it will take for half of the initial amount of the reactant to be converted into products.
For a first-order reaction, the half-life remains constant throughout the reaction and it will also be independent of the initial concentration of the reactant. Determining the half-lives is valuable in determining the time needed for a reactant to reach the desired level of transformation.
First-order half-life equation:
t½ = 0.693/k
where 0.693 is a constant derived from the natural logarithm of 2, and then divided by the rate constant (k) of the first-order reaction.
This equation is derived from the integrated rate law of a first-order reaction, i.e., ln ([A]t/[A]₀)= -kt. When the reactant concentration ([A]) is reduced to half of its initial value, it yields [A] = 0.5[A]₀. Substituting these values into the integrated rate law equation: ln(0.5) = -kt1/2. Simplifying further: t1/2 = (ln(2) / k) or t½ = 0.693/k.
Frequently Asked Questions – Faqs
What are the characteristics of first-order kinetics?
First-order kinetics or first-order reactions have the following characteristics that distinguish them from other kinetic models:
- Rate Dependence on a Single Reactant. The rate is directly proportional to the concentration of a single reactant. For example, increasing the concentration increases the reaction rate.
- Exponential decay. The reactant concentration decreases exponentially over time, such that the rate of decay is gradual and proportional to the concentration at any given time.
- Constant Half-Life. Regardless of the initial concentration, the time it takes for the concentration to reduce by half remains the same.
- Dependence on Rate Constant. The rate constant determines the speed at which the reaction proceeds. A larger value indicates a faster reaction; a smaller value indicates a slower reaction.
- Linear Relationship in ln([A]) vs. time Plot. The natural logarithm of the concentration graphically plotted against time results in a straight line with a negative slope (-k).
What are the units of the rate constant for a first-order reaction?
The first-order reaction units will depend on the overall order of the reaction. In particular, the units for k (rate constants) are typically expressed as reciprocal time (t⁻¹) or inverse seconds (s⁻¹). This is so considering the integrated rate law equation for the first-order reaction, ln ([A]t/[A]₀)= -kt, where the units of the left side are the same as the units of the concentration, thus, the units of the right side will cancel out the units of concentration.
What is a pseudo-first-order reaction?
A pseudo-first-order reaction behaves like a first-order reaction because the rate equation of the reaction is expressed as a first-order rate equation. In reality, the reaction is a higher order because the reaction involves more than one reactant (remember that a true first-order reaction has a reaction rate directly proportional to the concentration of only one reactant). However, because one of the reactants is present in excess, its concentration is seemingly unchanged throughout the reaction. Thus, the reaction, when it depends on the other reactant concentration, then the result is apparently following a first-order rate equation.
NOTE IT!
Radioactive Decay and Archaeological Dating
First-order kinetics play a significant role in radioactive decay, a natural process where unstable atomic nuclei spontaneously break down and transform into more stable forms. The decay of radioactive isotopes, such as carbon-14 (C-14) and uranium-238 (U-238), follows first-order kinetics. This property has enabled scientists to develop the technique of radiocarbon dating, used to determine the age of ancient artifacts and archaeological remains. By measuring the ratio of C-14 to its stable isotopes in organic materials, scientists can estimate the time that has passed since the organism’s death. The predictable decay of radioactive isotopes exemplifies the consistent and mathematical nature of first-order kinetics.
Watch this vid about first-order kinetics:
Take the First Order Kinetics – Biology Quiz!
References
- Atkins, P., & de Paula, J. (2010). Atkins’ Physical Chemistry. Oxford University Press.
- Laidler, K. J. (1987). Chemical Kinetics. Harper & Row.
- Levine, I. N. (2009). Physical Chemistry. McGraw-Hill.
- Nelson, D. L., & Cox, M. M. (2017). Lehninger Principles of Biochemistry. W. H. Freeman and Company.
- Silbey, R. J., Alberty, R. A., & Bawendi, M. G. (2017). Physical Chemistry. Wiley.
- Nelson, D. L., Cox, M. M., Lehninger, A. L., & Cox, M. M. (2008). Lehninger Principles of Biochemistry. W. H. Freeman.
- Berg, J. M., Tymoczko, J. L., & Gatto, G. J. (2014). Stryer’s Biochemistry. W. H. Freeman.
- Cantor, C. R., & Schimmel, P. R. (2014). Biophysical Chemistry: Principles and Techniques. W. H. Freeman.
- Giese, B. (2002). Molecular Mechanisms of Photosynthesis. John Wiley & Sons.
- Baynes, J. W., & Dominiczak, M. H. (2018). Medical Biochemistry. Elsevier.
- First-order Reaction: Definition, Examples, and Equations. (2023, May 3). Chemistry Learner. https://www.chemistrylearner.com/chemical-reactions/first-order-reaction
- 5.4: The Kinetics of Enzymatic Catalysis. (2020, July 15). Chemistry LibreTexts. https://chem.libretexts.org/Courses/Brevard_College/CHE_301_Biochemistry/05%3A_Enzymes/5.04%3A_The_Kinetics_of_Enzymatic_Catalysis
- First Order Reaction. (2014). Understanding First Order Reactions: Formulas & Examples. StudySmarter UK. https://www.studysmarter.co.uk/explanations/chemistry/kinetics/first-order-reaction/
- 14.3: Concentration and Rates (Differential Rate Laws). (2015, February 6). Chemistry LibreTexts. https://chem.libretexts.org/Bookshelves/General_Chemistry/Map%3A_Chemistry_-_The_Central_Science_(Brown_et_al.)/14%3A_Chemical_Kinetics/14.03%3A_Concentration_and_Rates_(Differential_Rate_Laws)#:~:text=A%20differential%20rate%20law%20expresses,specific%20time%20interval%20(%CE%94t).
©BiologyOnline.com. Content provided and moderated by Biology Online Editors.